Tides and Water Levels
What Causes Tides?
Gravity is one major force that creates tides. In 1687, Sir Isaac Newton explained that ocean tides result from the gravitational attraction of the sun and moon on the oceans of the earth (Sumich, J.L., 1996).
Newton’s law of universal gravitation states that the gravitational attraction between two bodies is directly proportional to their masses, and inversely proportional to the square of the distance between the bodies (Sumich, J.L., 1996; Thurman, H.V., 1994). Therefore, the greater the mass of the objects and the closer they are to each other, the greater the gravitational attraction between them (Ross, D.A. 1995).
Tidal forces are based on the gravitational attractive force. With regard to tidal forces on the Earth, the distance between two objects usually is more critical than their masses. Tidal generating forces vary inversely as the cube of the distance from the tide generating object. Gravitational attractive forces only vary inversely to the square of the distance between the objects (Thurman, H.V., 1994). The effect of distance on tidal forces is seen in the relationship between the sun, the moon, and the Earth’s waters.
Our sun is 27 million times larger than our moon. Based on its mass, the sun's gravitational attraction to the Earth is more than 177 times greater than that of the moon to the Earth. If tidal forces were based solely on comparative masses, the sun should have a tide-generating force that is 27 million times greater than that of the moon. However, the sun is 390 times further from the Earth than is the moon. Thus, its tide-generating force is reduced by 3903, or about 59 million times less than the moon. Because of these conditions, the sun’s tide-generating force is about half that of the moon (Thurman, H.V., 1994).
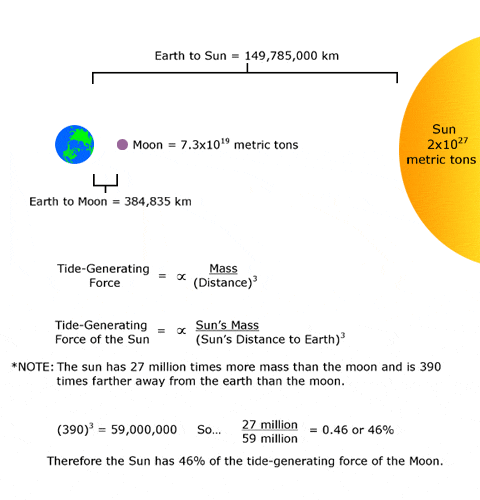
The relationship between the masses of the Earth, moon and sun and their distances to each other play a critical role in affecting the Earth's tides. Although the sun is 27 million times more massive than the moon, it is 390 times further away from the Earth than the moon. Tidal generating forces vary inversely as the cube of the distance from the tide-generating object. This means that the sun’s tidal generating force is reduced by 3903 (about 59 million times) compared to the tide-generating force of the moon. Therefore, the sun’s tide-generating force is about half that of the moon, and the moon is the dominant force affecting the Earth’s tides.
Tides and Water Levels
